1. Tổng hai vectơ
a) Định nghĩa: Cho hai vectơ \(\vec a\), \(\vec b.\) Từ điểm \(A\) tùy ý vẽ \(\overrightarrow {AB} = \overrightarrow a \) rồi từ \(B\) vẽ \(\overrightarrow {BC} = \overrightarrow b \), khi đó vectơ \(\overrightarrow {AC} \) được gọi là tổng của hai vectơ \(\overrightarrow a \), \(\overrightarrow b .\)
Kí hiệu \(\overrightarrow {AC} = \overrightarrow a + \overrightarrow b .\)
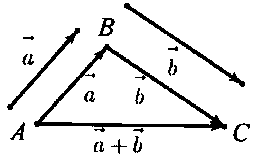
b) Tính chất
+ Giao hoán: \(\vec a + \vec b = \vec b + \vec a.\)
+ Kết hợp: \((\vec a + \vec b) + \vec c = \vec a + (\vec b + \vec c).\)
+ Tính chất vectơ-không: \(\vec a + \vec 0 = \vec a\), \(\forall \vec a.\)
2. Hiệu hai vectơ
a) Vectơ đối của một vectơ
Vectơ đối của vectơ \(\overrightarrow a \) là vectơ ngược hướng và cùng độ dài với vectơ \(\overrightarrow a .\)
Kí hiệu \( – \vec a.\)
Như vậy \(\vec a + ( – \vec a) = \vec 0\), \(\forall \vec a\) và \(\overrightarrow {AB} = – \overrightarrow {BA} .\)
b) Định nghĩa hiệu hai vectơ
Hiệu của hai vectơ \(\overrightarrow a \) và \(\overrightarrow b \) là tổng của vectơ \(\overrightarrow a \) và vectơ đối của vectơ \(\overrightarrow b .\) Kí hiệu là \(\vec a – \vec b = \vec a + ( – \vec b).\)
3. Các quy tắc
Quy tắc ba điểm: Cho \(A\), \(B\), \(C\) tùy ý, ta có: \(\overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} .\)
Quy tắc hình bình hành: Nếu \(ABCD\) là hình bình hành thì \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC} .\)
Quy tắc về hiệu vectơ: Cho \(O\), \(A\), \(B\) tùy ý ta có: \(\overrightarrow {OB} – \overrightarrow {OA} = \overrightarrow {AB} .\)
Chú ý: Ta có thể mở rộng quy tắc ba điểm cho \(n\) điểm \({A_1}\), \({A_2}\), …, \({A_n}\):
\(\overrightarrow {{A_1}{A_2}} + \overrightarrow {{A_2}{A_3}} + \ldots + \overrightarrow {{A_{n – 1}}{A_n}} = \overrightarrow {{A_1}{A_n}} .\)
B. CÁC DẠNG TOÁN VÀ PHƯƠNG PHÁP GIẢI
DẠNG TOÁN 1: XÁC ĐỊNH ĐỘ DÀI TỔNG, HIỆU CỦA CÁC VECTƠ.
1. PHƯƠNG PHÁP GIẢI
Để xác định độ dài tổng và hiệu của các vectơ:
+ Trước tiên sử dụng định nghĩa về tổng, hiệu hai vectơ và các tính chất, quy tắc để xác định phép toán vectơ đó.
+ Dựa vào tính chất của hình, sử dụng định lí Pitago, hệ thức lượng trong tam giác vuông để xác định độ dài vectơ đó.
2. CÁC VÍ DỤ
Ví dụ 1: Cho tam giác \(ABC\) vuông tại \(A\) có \(\widehat {ABC} = {30^0}\) và \(BC = a\sqrt 5 .\) Tính độ dài của các vectơ \(\overrightarrow {AB} + \overrightarrow {BC} \), \(\overrightarrow {AC} – \overrightarrow {BC} \) và \(\overrightarrow {AB} + \overrightarrow {AC} .\)
Theo quy tắc ba điểm ta có:
\(\overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} .\)
Mà \(\sin \widehat {ABC} = \frac{{AC}}{{BC}}.\)
\( \Rightarrow AC = BC.\sin \widehat {ABC}\) \( = a\sqrt 5 .\sin {30^0} = \frac{{a\sqrt 5 }}{2}.\)
Do đó \(|\overrightarrow {AB} + \overrightarrow {BC} | = |\overrightarrow {AC} |\) \( = AC = \frac{{a\sqrt 5 }}{2}.\)
\(\overrightarrow {AC} – \overrightarrow {BC} = \overrightarrow {AC} + \overrightarrow {CB} = \overrightarrow {AB} .\)
Ta có \(A{C^2} + A{B^2} = B{C^2}\) \( \Rightarrow AB = \sqrt {B{C^2} – A{C^2}} \) \( = \sqrt {5{a^2} – \frac{{5{a^2}}}{4}} = \frac{{a\sqrt {15} }}{2}.\)
Vì vậy \(|\overrightarrow {AC} – \overrightarrow {BC} | = |\overrightarrow {AB} |\) \( = AB = \frac{{a\sqrt {15} }}{2}.\)
Gọi \(D\) là điểm sao cho tứ giác \(ABDC\) là hình bình hành.
Khi đó theo quy tắc hình bình hành ta có \(\overrightarrow {AB} + \overrightarrow {AC} = \overrightarrow {AD} .\)
Vì tam giác \(ABC\) vuông ở \(A\) nên tứ giác \(ABDC\) là hình chữ nhật, suy ra \(AD = BC = a\sqrt 5 .\)
Vậy \(|\overrightarrow {AB} + \overrightarrow {AC} | = |\overrightarrow {AD} | = AD = a\sqrt 5 .\)
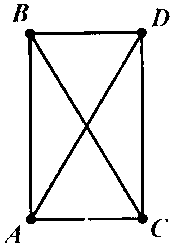
Ví dụ 2: Cho hình vuông \(ABCD\) có tâm là \(O\) và cạnh \(a.\) \(M\) là một điểm bất kỳ.
a) Tính \(|\overrightarrow {AB} + \overrightarrow {AD} |\), \(|\overrightarrow {OA} – \overrightarrow {BO} |\), \(|\overrightarrow {CD} – \overrightarrow {DA} |.\)
b) Chứng minh rằng \(\overrightarrow u = \overrightarrow {MA} + \overrightarrow {MB} – \overrightarrow {MC} – \overrightarrow {MD} \) không phụ thuộc vị trí điểm \(M.\) Tính độ dài vectơ \(\overrightarrow u .\)
a) Theo quy tắc hình bình hành ta có \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC} .\)
Suy ra \(|\overrightarrow {AB} + \overrightarrow {AD} | = |\overrightarrow {AC} | = AC.\)
Áp dụng định lí Pitago ta có:
\(A{C^2} = A{B^2} + B{C^2} = 2{a^2}\) \( \Rightarrow AC = \sqrt 2 a.\)
Vậy \(|\overrightarrow {AB} + \overrightarrow {AD} | = a\sqrt 2 .\)
Vì \(O\) là tâm của hình vuông nên \(\overrightarrow {OA} = \overrightarrow {CO} \), suy ra \(\overrightarrow {OA} – \overrightarrow {CB} = \overrightarrow {CO} – \overrightarrow {BO} = \overrightarrow {CB} .\)
Vậy \(|\overrightarrow {OA} – \overrightarrow {BO} | = |\overrightarrow {CB} | = a.\)
Do \(ABCD\) là hình vuông nên \(\overrightarrow {CD} = \overrightarrow {BA} \), suy ra \(\overrightarrow {CD} – \overrightarrow {DA} = \overrightarrow {BA} + \overrightarrow {AD} = \overrightarrow {BD} .\)
Mà \(|\overrightarrow {BD} | = BD = \sqrt {A{B^2} + A{D^2}} = a\sqrt 2 \) suy ra \(|\overrightarrow {CD} – \overrightarrow {DA} | = a\sqrt 2 .\)
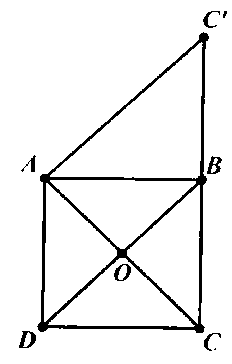
b) Theo quy tắc phép trừ ta có: \(\overrightarrow u = (\overrightarrow {MA} – \overrightarrow {MC} ) + (\overrightarrow {MB} – \overrightarrow {MD} )\) \( = \overrightarrow {CA} + \overrightarrow {DB} .\)
Suy ra \(\overrightarrow u \) không phụ thuộc vị trí điểm \(M.\)
Qua \(A\) kẻ đường thẳng song song với \(DB\) cắt \(BC\) tại \(C’.\)
Khi đó tứ giác \(ADBC’\) là hình bình hành (vì có cặp cạnh đối song song) suy ra \(\overrightarrow {DB} = \overrightarrow {AC’} .\)
Do đó \(\overrightarrow u = \overrightarrow {CA} + \overrightarrow {AC’} = \overrightarrow {CC’} .\)
Vì vậy \(|\vec u| = \left| {\overrightarrow {CC’} } \right| = BC + BC’\) \( = a + a = 2a.\)
3. BÀI TẬP LUYỆN TẬP
Bài 1: Cho tam giác \(ABC\) đều cạnh \(a.\) Tính độ dài của các vectơ sau \(\overrightarrow {AB} – \overrightarrow {AC} \), \(\overrightarrow {AB} + \overrightarrow {AC} .\)
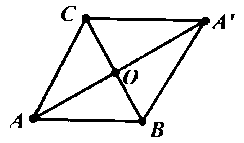
Theo quy tắc phép trừ ta có:
\(\overrightarrow {AB} – \overrightarrow {AC} = \overrightarrow {CB} \) \( \Rightarrow |\overrightarrow {AB} – \overrightarrow {AC} | = BC = a.\)
Gọi \(A’\) là đỉnh của hình bình hành \(ABA’C\) và \(O\) là tâm hình bình hành đó.
Khi đó ta có \(\overrightarrow {AB} + \overrightarrow {AC} = \overrightarrow {AA’} .\)
Ta có \(AO = \sqrt {A{B^2} – O{B^2}} \) \( = \sqrt {{a^2} – \frac{{{a^2}}}{4}} = \frac{{a\sqrt 3 }}{2}.\)
Suy ra \(|\overrightarrow {AB} + \overrightarrow {AC} | = AA’ = 2AO = a\sqrt 3 .\)
Bài 2: Cho hình vuông \(ABCD\) có tâm là \(O\) và cạnh \(a.\) \(M\) là một điểm bất kỳ.
a) Tính \(|\overrightarrow {AB} + \overrightarrow {OD} |\), \(|\overrightarrow {AB} – \overrightarrow {OC} + \overrightarrow {OD} |.\)
b) Tính độ dài vectơ \(\overrightarrow {MA} – \overrightarrow {MB} – \overrightarrow {MC} + \overrightarrow {MD} .\)
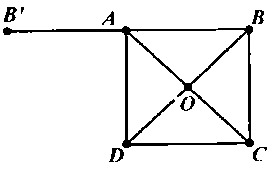
a) Ta có: \(\overrightarrow {OD} = \overrightarrow {BO} \) \( \Rightarrow \overrightarrow {AB} + \overrightarrow {OD} = \overrightarrow {AB} + \overrightarrow {BO} = \overrightarrow {AO} .\)
\(|\overrightarrow {AB} + \overrightarrow {OD} | = AO = \frac{{AC}}{2} = \frac{{a\sqrt 2 }}{2}.\)
Ta có \(\overrightarrow {OC} = \overrightarrow {AO} \) suy ra:
\(\overrightarrow {AB} – \overrightarrow {OC} + \overrightarrow {OD} \) \( = \overrightarrow {AB} – \overrightarrow {AO} + \overrightarrow {OD} \) \( = \overrightarrow {OB} + \overrightarrow {OD} = \vec 0.\)
\( \Rightarrow |\overrightarrow {AB} – \overrightarrow {OC} + \overrightarrow {OD} | = 0.\)
b) Áp dụng quy tắc phép trừ ta có:
\(\overrightarrow {MA} – \overrightarrow {MB} – \overrightarrow {MC} + \overrightarrow {MD} \) \( = (\overrightarrow {MA} – \overrightarrow {MB} ) – (\overrightarrow {MC} – \overrightarrow {MD} )\) \( = \overrightarrow {BA} – \overrightarrow {DC} .\)
Lấy \(B’\) là điểm đối xứng của \(B\) qua \(A.\)
Khi đó \( – \overrightarrow {DC} = \overrightarrow {AB’} \) \( \Rightarrow \overrightarrow {BA} – \overrightarrow {DC} \) \( = \overrightarrow {BA} + \overrightarrow {AB’} = \overrightarrow {BB’} .\)
Suy ra \(|\overrightarrow {MA} – \overrightarrow {MB} – \overrightarrow {MC} + \overrightarrow {MD} |\) \( = |\overrightarrow {BB’} |\) \( = BB’ = 2a.\)
Bài 3: Cho hình thoi \(ABCD\) cạnh \(a\) và \(\widehat {BCD} = {60^0}.\) Gọi \(O\) là tâm hình thoi. Tính \(|\overrightarrow {AB} + \overrightarrow {AD} |\), \(|\overrightarrow {OB} – \overrightarrow {DC} |.\)
Ta có:
\(|\overrightarrow {AB} + \overrightarrow {AD} | = |\overrightarrow {AC} |\) \( = 2a\cos {30^0} = a\sqrt 3 .\)
\(|\overrightarrow {OB} – \overrightarrow {DC} | = |\overrightarrow {CO} |\) \( = a\cos {30^0} = \frac{{a\sqrt 3 }}{2}.\)
Bài 4: Cho bốn điểm \(A\), \(B\), \(C\), \(O\) phân biệt có độ dài ba vectơ \(\overrightarrow {OA} \), \(\overrightarrow {OB} \), \(\overrightarrow {OC} \) cùng bằng \(a\) và \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} = \vec 0.\)
a) Tính các góc \(\widehat {AOB}\), \(\widehat {BOC}\), \(\widehat {COA}.\)
b) Tính \(|\overrightarrow {OB} + \overrightarrow {AC} – \overrightarrow {OA} |.\)
a) Từ giả thiết suy ra ba điểm \(A\), \(B\), \(C\) tạo thành tam giác đều nhận \(O\) làm trọng tâm, do đó: \(\widehat {AOB} = \widehat {BOC} = \widehat {COA} = {120^0}.\)
b) Gọi \(I\) là trung điểm \(BC.\)
Theo câu a ta có \(\Delta ABC\) đều nên \(AI = \frac{{\sqrt 3 }}{2}a.\)
\(|\overrightarrow {OB} + \overrightarrow {AC} – \overrightarrow {OA} | = a\sqrt 3 .\)
Bài 5: Cho góc \(\widehat {Oxy}\). Trên \(Ox\), \(Oy\) lấy hai điểm \(A\), \(B.\) Tìm điều kiện của \(A\), \(B\) sao cho \(\overrightarrow {OA} + \overrightarrow {OB} \) nằm trên phân giác của góc \(\widehat {Oxy}.\)
Dựng hình bình hành \(OACB.\)
Khi đó: \(\overrightarrow {OA} + \overrightarrow {OB} = \overrightarrow {OC} .\)
Vậy \(\overrightarrow {OC} \) nằm trên phân giác góc \(xOy \Leftrightarrow OACB\) là hình thoi \( \Leftrightarrow OA = OB.\)
DẠNG TOÁN 2: CHỨNG MINH ĐẲNG THỨC VECTƠ.
1. PHƯƠNG PHÁP GIẢI
Để chứng minh đẳng thức vectơ ta có các cách biến đổi:
+ Vế này thành vế kia.
+ Biến đổi tương đương.
+ Biến đổi hai vế cùng bằng một đại lượng trung gian.
Trong quá trình biến đổi ta cần sử dụng linh hoạt ba quy tắc tính vectơ.
Lưu ý: Khi biến đổi cần phải “hướng đích”, chẳng hạn biến đổi vế phải, ta cần xem vế trái có đại lượng nào để từ đó liên tưởng đến kiến thức đã có để làm sao xuất hiện các đại lượng ở vế trái. Và ta thường biến đổi vế phức tạp về vế đơn giản hơn.
2. CÁC VÍ DỤ
Ví dụ 1: Cho năm điểm \(A\), \(B\), \(C\), \(D\), \(E.\) Chứng minh rằng:
a) \(\overrightarrow {AB} + \overrightarrow {CD} + \overrightarrow {EA} = \overrightarrow {CB} + \overrightarrow {ED} .\)
b) \(\overrightarrow {AC} + \overrightarrow {CD} – \overrightarrow {EC} = \overrightarrow {AE} – \overrightarrow {DB} + \overrightarrow {CB} .\)
a) Biến đổi vế trái, ta có:
\(VT = (\overrightarrow {AC} + \overrightarrow {CB} ) + \overrightarrow {CD} + (\overrightarrow {ED} + \overrightarrow {DA} )\) \( = (\overrightarrow {CB} + \overrightarrow {ED} ) + (\overrightarrow {AC} + \overrightarrow {CD} ) + \overrightarrow {DA} \) \( = (\overrightarrow {CB} + \overrightarrow {ED} ) + \overrightarrow {AD} + \overrightarrow {DA} \) \( = \overrightarrow {CB} + \overrightarrow {ED} = VP.\)
b) Đẳng thức tương đương với:
\((\overrightarrow {AC} – \overrightarrow {AE} ) + (\overrightarrow {CD} – \overrightarrow {CB} )\) \( – \overrightarrow {EC} + \overrightarrow {DB} = \overrightarrow 0 \) \( \Leftrightarrow \overrightarrow {EC} + \overrightarrow {BD} – \overrightarrow {EC} + \overrightarrow {DB} = \vec 0\) \(\overrightarrow {BD} + \overrightarrow {DB} = \vec 0\) (đúng).
Suy ra điều phải chứng minh.
Ví dụ 2: Cho hình bình hành \(ABCD\) tâm \(O.\) \(M\) là một điểm bất kì trong mặt phẳng. Chứng minh rằng:
a) \(\overrightarrow {BA} + \overrightarrow {DA} + \overrightarrow {AC} = \vec 0.\)
b) \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \vec 0.\)
c) \(\overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} .\)
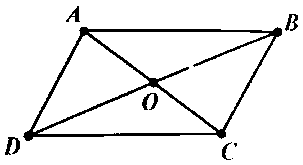
a) Ta có: \(\overrightarrow {BA} + \overrightarrow {DA} + \overrightarrow {AC} \) \( = – \overrightarrow {AB} – \overrightarrow {AD} + \overrightarrow {AC} \) \( = – (\overrightarrow {AB} + \overrightarrow {AD} ) + \overrightarrow {AC} .\)
Theo quy tắc hình bình hành ta có: \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC} .\)
Suy ra: \(\overrightarrow {BA} + \overrightarrow {DA} + \overrightarrow {AC} \) \( = – \overrightarrow {AC} + \overrightarrow {AC} = \vec 0.\)
b) Vì \(ABCD\) là hình bình hành nên ta có:
\(\overrightarrow {OA} = \overrightarrow {CO} \) \( \Rightarrow \overrightarrow {OA} + \overrightarrow {OC} = \overrightarrow {OA} + \overrightarrow {AO} = \vec 0.\)
Tương tự: \(\overrightarrow {OB} + \overrightarrow {OD} = \vec 0\) \( \Rightarrow \overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \vec 0.\)
c)
Cách 1: Vì \(ABCD\) là hình bình hành nên \(\overrightarrow {AB} = \overrightarrow {DC} \) \( \Rightarrow \overrightarrow {BA} + \overrightarrow {DC} = \overrightarrow {BA} + \overrightarrow {AB} = \vec 0.\)
\( \Rightarrow \overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {BA} + \overrightarrow {MD} + \overrightarrow {DC} \) \( = \overrightarrow {MB} + \overrightarrow {MD} + \overrightarrow {BA} + \overrightarrow {DC} \) \( = \overrightarrow {MB} + \overrightarrow {MD} .\)
Cách 2: Đẳng thức tương đương với:
\(\overrightarrow {MA} – \overrightarrow {MB} = \overrightarrow {MD} – \overrightarrow {MC} \) \( \Leftrightarrow \overrightarrow {BA} = \overrightarrow {CD} \) (đúng do \(ABCD\) là hình bình hành).
Ví dụ 3: Cho tam giác \(ABC.\) Gọi \(M\), \(N\), \(P\) lần lượt là trung điểm của \(BC\), \(CA\), \(AB.\) Chứng minh rằng:
a) \(\overrightarrow {BM} + \overrightarrow {CN} + \overrightarrow {AP} = \overrightarrow 0 .\)
b) \(\overrightarrow {AP} + \overrightarrow {AN} – \overrightarrow {AC} + \overrightarrow {BM} = \vec 0.\)
c) \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} = \overrightarrow {OM} + \overrightarrow {ON} + \overrightarrow {OP} \) với \(O\) là điểm bất kì.
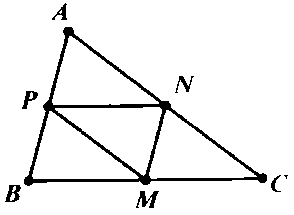
a) Vì \(PN\), \(MN\) là đường trung bình của tam giác \(ABC\) nên \(PN // BM\), \(MN // BP\) suy ra tứ giác \(BMNP\) là hình bình hành.
\( \Rightarrow \overrightarrow {BM} = \overrightarrow {PN} .\)
\(N\) là trung điểm của \(AC\) \( \Rightarrow \overrightarrow {CN} = \overrightarrow {NA} .\)
Do đó theo quy tắc ba điểm ta có:
\(\overrightarrow {BM} + \overrightarrow {CN} + \overrightarrow {AP} \) \( = (\overrightarrow {PN} + \overrightarrow {NA} ) + \overrightarrow {AP} \) \( = \overrightarrow {PA} + \overrightarrow {AP} = \vec 0.\)
b) Vì tứ giác \(APMN\) là hình bình hành nên theo quy tắc hình bình hành, ta có: \(\overrightarrow {AP} + \overrightarrow {AN} = \overrightarrow {AM} .\)
Kết hợp với quy tắc phép trừ \( \Rightarrow \overrightarrow {AP} + \overrightarrow {AN} – \overrightarrow {AC} + \overrightarrow {BM} \) \( = \overrightarrow {AM} – \overrightarrow {AC} + \overrightarrow {BM} \) \( = \overrightarrow {CM} + \overrightarrow {BM} .\)
Mà \(\overrightarrow {CM} + \overrightarrow {BM} = \vec 0\) do \(M\) là trung điểm của \(BC.\)
Vậy \(\overrightarrow {AP} + \overrightarrow {AN} – \overrightarrow {AC} + \overrightarrow {BM} = \vec 0.\)
c) Theo quy tắc ba điểm, ta có:
\(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} \) \( = (\overrightarrow {OP} + \overrightarrow {PA} )\) \( + (\overrightarrow {OM} + \overrightarrow {MB} )\) \( + (\overrightarrow {ON} + \overrightarrow {NC} )\) \( = (\overrightarrow {OM} + \overrightarrow {ON} + \overrightarrow {OP} )\) \( + \overrightarrow {PA} + \overrightarrow {MB} + \overrightarrow {NC} \) \( = (\overrightarrow {OM} + \overrightarrow {ON} + \overrightarrow {OP} )\) \( – (\overrightarrow {BM} + \overrightarrow {CN} + \overrightarrow {AP} ).\)
Theo câu a ta có \(\overrightarrow {BM} + \overrightarrow {CN} + \overrightarrow {AP} = \overrightarrow 0 \), suy ra \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} = \overrightarrow {OM} + \overrightarrow {ON} + \overrightarrow {OP} .\)
3. BÀI TẬP LUYỆN TẬP
Bài 1: Cho bốn điểm \(A\), \(B\), \(C\), \(D.\) Chứng minh rằng:
a) \(\overrightarrow {DA} – \overrightarrow {CA} = \overrightarrow {DB} – \overrightarrow {CB} .\)
b) \(\overrightarrow {AC} + \overrightarrow {DA} + \overrightarrow {BD} = \overrightarrow {AD} – \overrightarrow {CD} + \overrightarrow {BA} .\)
a) Áp dụng quy tắc phép trừ, ta có:
\(\overrightarrow {DA} – \overrightarrow {CA} = \overrightarrow {DB} – \overrightarrow {CB} \) \( \Leftrightarrow \overrightarrow {DA} – \overrightarrow {DB} = \overrightarrow {CA} – \overrightarrow {CB} \) \( \Leftrightarrow \overrightarrow {BA} = \overrightarrow {BA} \) (đúng).
b) Áp dụng quy tắc ba điểm, ta có:
\(\overrightarrow {AC} + \overrightarrow {DA} + \overrightarrow {BD} \) \( = \overrightarrow {AD} – \overrightarrow {CD} + \overrightarrow {BA} \) \( \Leftrightarrow (\overrightarrow {DA} + \overrightarrow {AC} ) + \overrightarrow {BD} \) \( = (\overrightarrow {BA} + \overrightarrow {AD} ) – \overrightarrow {CD} \) \( \Leftrightarrow \overrightarrow {DC} + \overrightarrow {BD} = \overrightarrow {BD} – \overrightarrow {CD} \) (đúng).
Bài 2: Cho các điểm \(A\), \(B\), \(C\), \(D\), \(E\), \(F.\) Chứng minh rằng \(\overrightarrow {AD} + \overrightarrow {BE} + \overrightarrow {CF} = \overrightarrow {AE} + \overrightarrow {BF} + \overrightarrow {CD} .\)
Cách 1: Đẳng thức cần chứng minh tương đương với:
\((\overrightarrow {AD} – \overrightarrow {AE} ) + (\overrightarrow {BE} – \overrightarrow {BF} )\) \( + (\overrightarrow {CF} – \overrightarrow {CD} ) = \vec 0.\)
\( \Leftrightarrow \overrightarrow {ED} + \overrightarrow {FE} + \overrightarrow {DF} = \vec 0\) \( \Leftrightarrow \overrightarrow {EF} + \overrightarrow {FE} = \vec 0\) (đúng).
Cách 2: \(VT = \overrightarrow {AD} + \overrightarrow {BE} + \overrightarrow {CF} \) \( = (\overrightarrow {AE} + \overrightarrow {ED} ) + (\overrightarrow {BF} + \overrightarrow {FE} ) + (\overrightarrow {CD} + \overrightarrow {DF} )\) \( = \overrightarrow {AE} + \overrightarrow {BF} + \overrightarrow {CD} + \overrightarrow {ED} + \overrightarrow {FE} + \overrightarrow {DF} \) \( = \overrightarrow {AE} + \overrightarrow {BF} + \overrightarrow {CD} = VP.\)
Bài 3: Cho hình bình hành \(ABCD\) tâm \(O.\) \(M\) là một điểm bất kì trong mặt phẳng. Chứng minh rằng:
a) \(\overrightarrow {AB} + \overrightarrow {OD} + \overrightarrow {OC} = \overrightarrow {AC} .\)
b) \(\overrightarrow {BA} + \overrightarrow {BC} + \overrightarrow {OB} = \overrightarrow {OD} .\)
c) \(\overrightarrow {BA} + \overrightarrow {BC} + \overrightarrow {OB} = \overrightarrow {MO} – \overrightarrow {MB} .\)
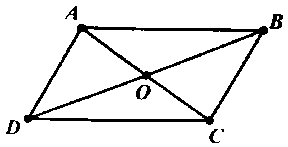
a) Ta có \(\overrightarrow {OD} = \overrightarrow {BO} \) do đó:
\(\overrightarrow {AB} + \overrightarrow {OD} + \overrightarrow {OC} \) \( = \overrightarrow {AB} + \overrightarrow {BO} + \overrightarrow {OC} \) \( = \overrightarrow {AO} + \overrightarrow {OC} = \overrightarrow {AC} .\)
b) Theo quy tắc hình bình hành, ta có:
\(\overrightarrow {BA} + \overrightarrow {BC} + \overrightarrow {OB} \) \( = \overrightarrow {BD} + \overrightarrow {OB} \) \( = \overrightarrow {OB} + \overrightarrow {BD} = \overrightarrow {OD} .\)
c) Theo câu b ta có: \(\overrightarrow {BA} + \overrightarrow {BC} + \overrightarrow {OB} = \overrightarrow {OD} .\)
Theo quy tắc trừ ta có: \(\overrightarrow {MO} – \overrightarrow {MB} = \overrightarrow {BO} .\)
Mà \(\overrightarrow {OD} = \overrightarrow {BO} \) suy ra \(\overrightarrow {BA} + \overrightarrow {BC} + \overrightarrow {OB} = \overrightarrow {MO} – \overrightarrow {MB} .\)
Bài 4: Cho tam giác \(ABC.\) Gọi \(M\), \(N\), \(P\) lần lượt là trung điểm của \(BC\), \(CA\), \(AB.\) Chứng minh rằng:
a) \(\overrightarrow {NA} + \overrightarrow {PB} + \overrightarrow {MC} = \vec 0.\)
b) \(\overrightarrow {MC} + \overrightarrow {BP} + \overrightarrow {NC} = \overrightarrow {BC} .\)
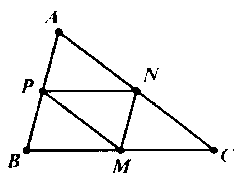
a) Vì \(\overrightarrow {PB} = \overrightarrow {AP} \), \(\overrightarrow {MC} = \overrightarrow {PN} \) nên: \(\overrightarrow {NA} + \overrightarrow {PB} + \overrightarrow {MC} \) \( = \overrightarrow {NA} + \overrightarrow {AP} + \overrightarrow {PN} \) \( = \overrightarrow {NP} + \overrightarrow {PN} = \vec 0.\)
b) Vì \(\overrightarrow {MC} = \overrightarrow {BM} \) và kết hợp với quy tắc ba điểm, quy tắc hình bình hành, ta có:
\(\overrightarrow {MC} + \overrightarrow {BP} + \overrightarrow {NC} \) \( = \overrightarrow {BM} + \overrightarrow {BP} + \overrightarrow {NC} \) \( = \overrightarrow {BN} + \overrightarrow {NC} = \overrightarrow {BC} .\)
Bài 5: Cho hai hình bình hành \(ABCD\) và \(AB’C’D’\) có chung đỉnh \(A.\) Chứng minh rằng: \(\overrightarrow {B’B} + \overrightarrow {CC’} + \overrightarrow {D’D} = \vec 0.\)
Theo quy tắc trừ và quy tắc hình bình hành, ta có:
\(\overrightarrow {B’B} + \overrightarrow {CC’} + \overrightarrow {D’D} \) \( = \left( {\overrightarrow {AB} – \overrightarrow {AB’} } \right) + \left( {\overrightarrow {AC’} – \overrightarrow {AC} } \right) + \left( {\overrightarrow {AD} – \overrightarrow {AD’} } \right)\) \( = \left( {\overrightarrow {AB} + \overrightarrow {AD} } \right) – \overrightarrow {AC} \) \( – \left( {\overrightarrow {AB’} + \overrightarrow {AD’} } \right) + \overrightarrow {AC’} = \overrightarrow 0 .\)
Bài 6: Cho ngũ giác đều \(ABCDE\) tâm \(O.\) Chứng minh rằng: \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OE} + \overrightarrow {OF} = \vec 0.\)
Đặt \(\overrightarrow u = \overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OE} + \overrightarrow {OF} .\)
Vì ngũ giác đều nên vectơ \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OE} \) cùng phương với \(\overrightarrow {OF} \) nên \(\overrightarrow u \) cùng phương với \(\overrightarrow {OF} .\)
Tương tự \(\overrightarrow u \) cùng phương với \(\overrightarrow {OE} .\)
Suy ra \(\overrightarrow u = \vec 0.\)
Bài 7: Cho hình bình hành \(ABCD.\) Dựng \(\overrightarrow {AM} = \overrightarrow {BA} \), \(\overrightarrow {MN} = \overrightarrow {DA} \), \(\overrightarrow {NP} = \overrightarrow {DC} \), \(\overrightarrow {PQ} = \overrightarrow {BC} .\)
Chứng minh rằng: \(\overrightarrow {AQ} = \overrightarrow 0 .\)
Theo quy tắc ba điểm, ta có: \(\overrightarrow {AQ} = \overrightarrow {AM} + \overrightarrow {MN} + \overrightarrow {NP} + \overrightarrow {PQ} \) \( = \overrightarrow {BA} + \overrightarrow {DA} + \overrightarrow {DC} + \overrightarrow {BC} .\)
Mặt khác \(\overrightarrow {BA} + \overrightarrow {BC} = \overrightarrow {BD} \), \(\overrightarrow {DA} + \overrightarrow {DC} = \overrightarrow {DB} .\)
Suy ra \(\overrightarrow {AQ} = \overrightarrow {BD} + \overrightarrow {DB} = \vec 0.\)